The method of symmetrical components simplifies the analysis of unbalanced three-phase power systems under normal and abnormal conditions. This method simplifies the problems of unbalanced three-phase systems and is often called a three-component or symmetrical components method.
The unbalanced three-phase system can be solved using symmetrical components, which can then be converted back to the actual circuit. The balanced components include a positive sequence component, a negative sequence component, and a zero-phase sequence component.
In the figure below, there is an unbalanced voltage phasor system. The system has three phasors named Va, Vb, and Vc. These phasors have phase sequences: Va, Vb, and Vc. The positive component of the system also has the same phase sequence as Va, Vb, and Vc. However, the negative components have a different phase sequence: Va, Vc, and Vb.

In the positive phase sequence component, there is a set of three phasors that are equal in magnitude and spaced 120° apart from each other. The phase sequence of positive phase sequence components is the same as of the original unbalanced phasors. You can see the positive sequence component of the unbalanced three-phase system below.
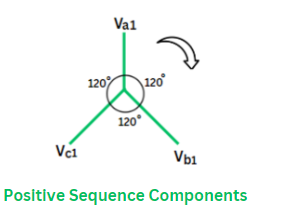
We represent positive sequence components as V1, I1, and Z1 for voltage, current, and impedance, respectively. The mathematical equations of these components are given below.
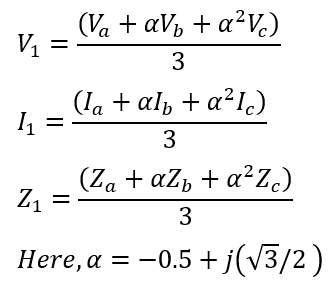
The negative phase sequence component has a set of three phasors. All three phasors are equal in magnitude, spaced 120° apart, and have a phase sequence opposite to the original phasors. This is depicted in the figure below.
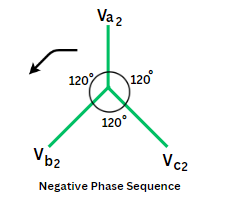
We represent negative sequence components as V2, I2, and Z2 for voltage, current, and impedance, respectively. The mathematical equations of these components are given below.
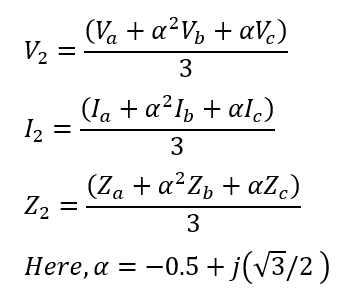
In zero sequence components, the three phasors have equal magnitude and zero phase displacement from each other. The figure below shows the zero sequence component.

We represent zero sequence components as V0, I0, and Z0 for voltage, current, and impedance, respectively. The mathematical equations of these components are given below.
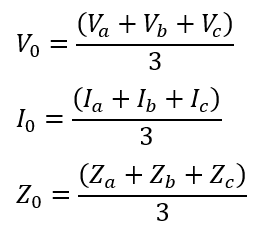
Zero and negative sequence components are absent from a three-phase balanced system.